Elements of Ellipse
Elements of the ellipse are shown in the figure below.
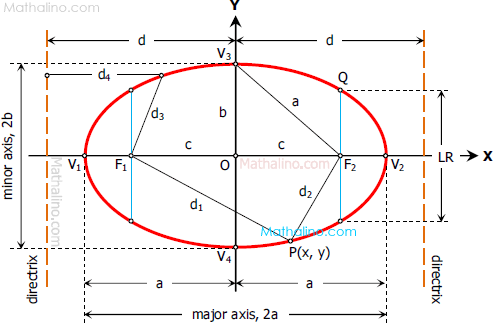
- Center (h, k). At the origin, (h, k) is (0, 0).
- Semi-major axis = a and semi-minor axis = b.
- Location of foci c, with respect to the center of ellipse. $c = \sqrt{a^2 - b^2}$.
- Length latus rectum, LR
 Consider the right triangle F1QF2:
Consider the right triangle F1QF2: - Eccentricity, e
$e = \dfrac{\text{distance from focus to ellipse}}{\text{distance from ellipse to directrix}}$
From the figure of the ellipse above,
$e = \dfrac{d_3}{d_4} = \dfrac{a}{d} = \dfrac{a - c}{d - a}$From
$\dfrac{a}{d} = \dfrac{a - c}{d - a}$$ad - a^2 = ad - cd$
$d = a^2 / c$
Thus,
$e = \dfrac{a}{d} = \dfrac{a}{a^2 / c}$$e = \dfrac{c}{a} \lt 1.0$ - Location of directrix d, with respect to the center of ellipse.
From the derivation of eccentricity,
$d = \dfrac{a}{e} \, \text{ or } d = \dfrac{a^2}{c}$
Based on the definition of ellipse
$z + \frac{1}{2}LR = 2a$
$z = 2a - \frac{1}{2}LR$
$z = \dfrac{4a - LR}{2}$
By Pythagorean Theorem
$(2c)^2 + (\frac{1}{2}LR)^2 = z^2$
$4c^2 + \frac{1}{4}(LR)^2 = \left( \dfrac{4a - LR}{2} \right)^2$
$4c^2 + \frac{1}{4}(LR)^2 = \dfrac{(4a - LR)^2}{4}$
$16c^2 + (LR)^2 = (4a - LR)^2$
$16c^2 + (LR)^2 = 16a^2 - 8a(LR) + (LR)^2$
$16c^2 = 16a^2 - 8a(LR)$
$8a(LR) = 16a^2 - 16c^2$
$LR = \dfrac{16a^2 - 16c^2}{8a}$
$LR = \dfrac{16(a^2 - c^2)}{8a}$
You can also find the same formula for the length of latus rectum of ellipse by using the definition of eccentricity.
- Add new comment
- 19525 reads
